Steel Beam Design – A Step-By-Step Guide
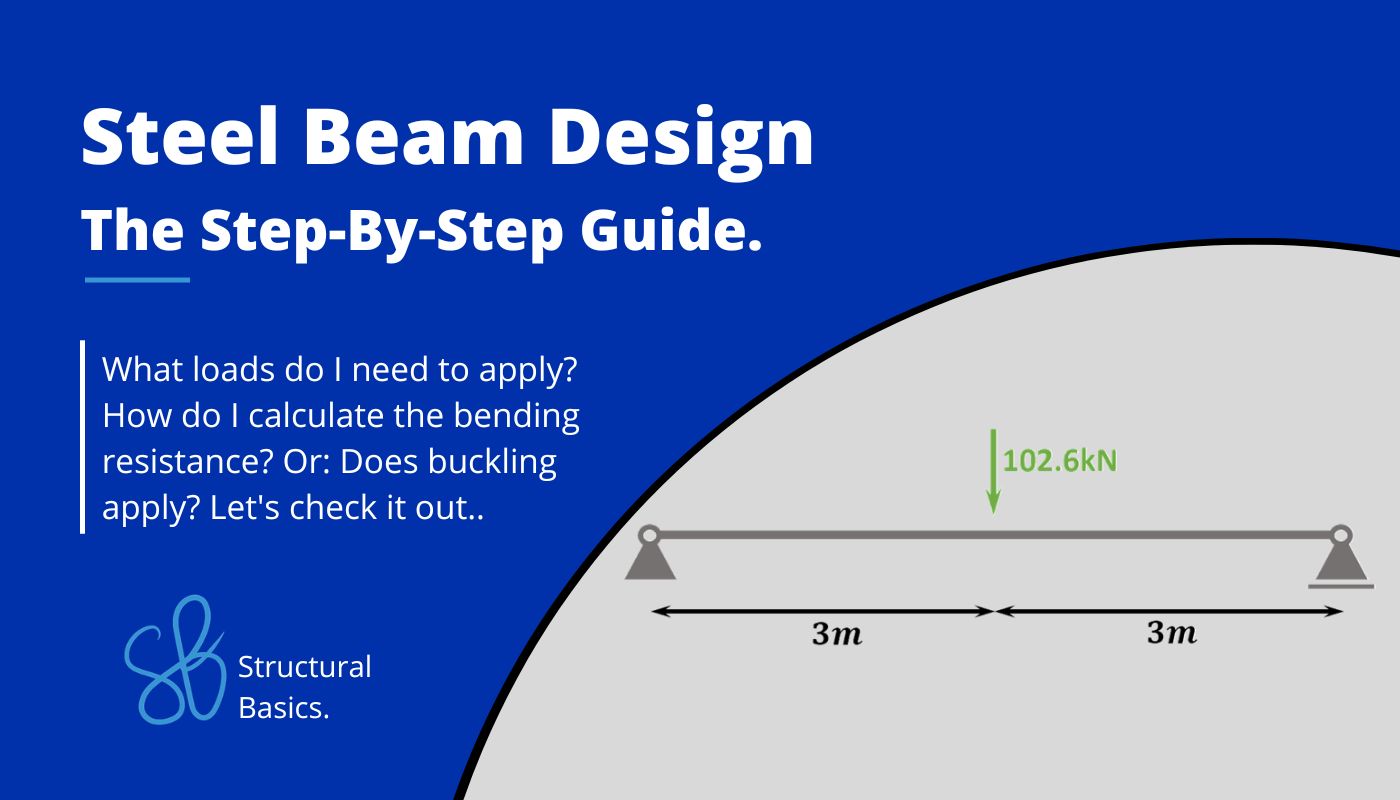
Designing steel beams is something structural engineering students learn early in university, as steel is one of the 3 most used structural building materials.
And beams are the most used static system – simply supported beams to be more precise.
Later on, structural engineers do steel beam design over and over.
So in this post we’ll show you, step-by step, how to design steel beams with a worked example, what loads can act on a beam and how to classify a steel Cross-section according to the Steel Eurocode EN 1993-1-1.
Not much more talk, let’s dive into it.
📃 Process of Steel Beam Design
Before we dive into the nerdy calculations, it’s good to get an overview of the steps that need to be taken to design a steel beam – in this case, a simply supported beam loaded with a point load.
- Calculate characteristic loads that act on the beam
- Load combinations
- Define properties of steel beam
- Cross-Section Classification
- Bending Verification
- Shear Verification
- Lateral Torsional Buckling Verification
- Deflection Verification
🙋♀️ Example Structures of Steel Beams
Steel beams are used in
- Warehouse structures
- Temporary event structures (tents, stages, etc.)
- Buildings
- Renovation projects as “reinforcement” of existing structure
- Factories
- Steel towers
to name a few.
The use of steel is also highly dependent on the country. In some countries steel structures are common, in some they’re not.
👆 Static System of Steel Beam
There is a variety of different static systems that the structural engineer can choose from, and often the beams act together with columns as frames.
Here is the static system we are gonna use in this tutorial.
Simply Supported Beam
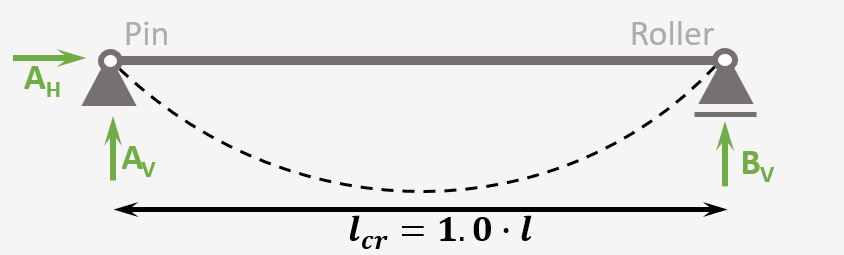
Support types
Roller & Pin
Reactions
Roller: Vertical
Pin: Vercial & Horizontal
Buckling length
$l_{cr} = 1.0 \cdot l$
It’s important to know about the different static systems, since each type of beam has a different buckling length, which influences the buckling resistance significantly❗
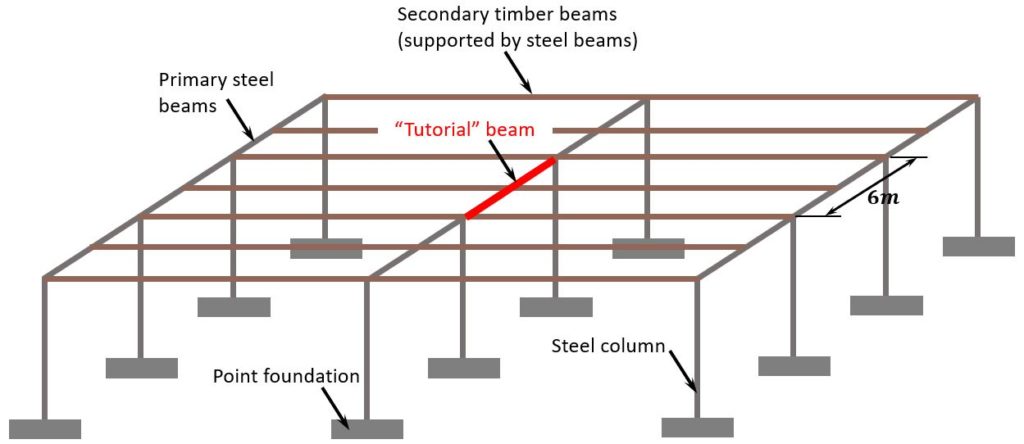
Now, let’s add some context to understand the overall statics of the building where the beam is part of.
The following picture shows where the steel beam could be used.
⬇️ Characteristic Loads of Steel Beam
The loads of a structure depend on its location, geometry, building type and other factors.
We’ll assume in this tutorial that we design the beam of a 1-storey building, such as a supermarket.
Now on a 1-storey building, the following loads act:
- Dead load of structural and non-structural elements
- Live load of the roof
- Snow load and
- Wind load
We’ll do a lot of assumptions in this tutorial, but if you want to learn more about loads on roofs, you can check out this article.
Load transfer
❗ The following load transfer explanation works if the building is a traditional structure with simply supported beams and columns❗
- Live, Snow, Wind and dead load (self-weight of roofing) are applied as area loads [kN/m2] on the roof.
The roof could for example be a trapezoidal roof sheet. This roof sheet transfers the loads to the secondary beams (in our case timber beams), which support the sheets. - By multiplying the area loads (live, snow, wind, dead load) with the spacing of the beams, line loads are calculated. These line load can now be applied to the secondary (timber) beams.
- The reaction force of the timber beam is then the characteristic vertical load that is applied at midspan to the primary steel beams.
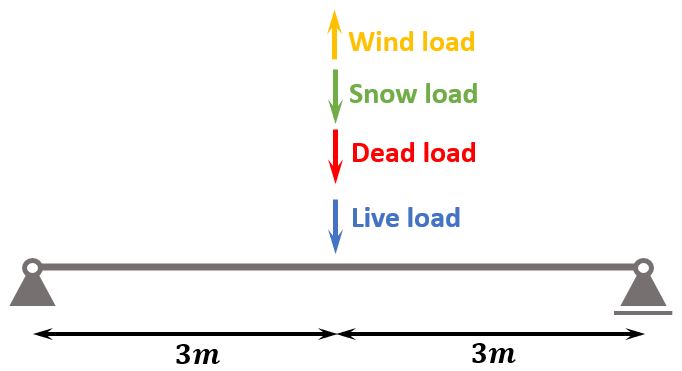
The following characteristic load values are assumptions.
| $g_{k}$ | 36 kN | Characteristic value of dead load |
| $q_{k}$ | 15 kN | Characteristic value of live load |
| $s_{k}$ | 30 kN | Characteristic value of snow load |
| $w_k$ | -4 kN | Characteristic value of wind load |
❗
The characteristic values of loads depend on a lot of different factors like location, National Annex and geometry of the building and roof to name just a few. Loads therefore need to be calculated for every structure.
➕ Load combinations
Load combinations combine several load cases and multiply the characteristic loads with safety factors.
Luckily, we have already written an extensive article about what load combinations are and how we use them.
In case you need to brush up on it or want to check how we derived the safety factors, you can read the blog post here.
ULS Load combinations
| LC1 | $1.35 * 36 kN $ | $48.6 kN$ | |
| LC2 | $1.35 * 36 kN + 1.5 * 15 kN$ | $71.1 kN$ | |
| LC3 | $1.35 * 36 kN + 1.5 * 15 kN + 0.7 * 1.5 * 30 kN$ | $102.6 kN$ | |
| LC4 | $1.35 * 36 kN + 0 * 1.5 * 15 kN + 1.5 * 30 kN$ | $93.6 kN$ | |
| LC5 | $1.35 * 36 kN + 1.5 * 15 kN + 0.7 * 1.5 * 30 kN + 0.6 * 1.5 * (-4 kN) $ | $99.0 kN$ | |
| LC6 | $1.35 * 36 kN + 0 * 1.5 * 15 kN + 1.5 * 30 kN + 0.6 * 1.5 * (-4 kN) $ | $90.0 kN$ | |
| LC7 | $1.35 * 36 kN + 0 * 1.5 * 15 kN + 0.7 * 1.5 * 30 kN + 1.5 * (-4 kN) $ | $74.1 kN$ | |
| LC8 | $1.35 * 36 kN + 1.5 * 30 kN $ | $93.6 kN$ | |
| LC9 | $1.35 * 36 kN + 1.5 * (-4 kN) $ | $42.6 kN$ | |
| LC10 | $1.35 * 36 kN + 1.5 * 15 kN + 0.6 * 1.5 * (-4 kN) $ | $67.5 kN$ | |
| LC11 | $1.35 * 36 kN + 1.5 * (-4 kN) + 0.7 * 1.5 * 30 kN $ | $74.1 kN$ | |
| LC12 | $1.35 * 36 kN + 1.5 * 15 kN + 0.6 * 1.5 * (-4 kN)$ | $67.5 kN$ |
SLS Load combinations
Now, we are a bit lazy with the SLS load combinations and only define the 2 combinations that can realistically be the leading one.
If you want to read more about the SLS load combinations, see a list of all LC’s and the safety factor values, then check out this article here.
| LC1 | $36 kN + 15 kN + 0.7 \cdot 30 kN$ | $72 kN$ | |
| LC2 | $36 kN +0 \cdot 15 kN + 30 kN$ | $66 kN$ |
👉 Steel Beam Properties
In this tutorial, we’ll design a steel beam which has the Cross-section of an IPE360. We’ll use the Cross-section properties from eurocodeapplied.com.
It’s btw a really good resource for all kind of steel cross-section properties.
We furthermore specify that the beam is of steel class S235.
Here are the geometrical properties of a IPE360 S235 Cross-section we are gonna use.
| Width | $b=170mm$ |
| Height | $h=360mm$ |
| Web thickness | $t_w = 8mm$ |
| Flange thickness | $t_f = 12.7mm$ |
| Root radius | $r = 18mm$ |
| Moment of inertia – strong axis | $I_y = 162.7 \cdot 10^6 mm^4$ |
| Moment of inertia – weak axis | $I_z = 10.43 \cdot 10^6 mm^4$ |
| Cross-sectional area | $A = 7273 mm^2$ |
| Plastic section modulus – strong axis | $W_{pl.y} = 1019 \cdot 10^3 mm^3$ |
| Torsion constant | $I_v = 370.8 \cdot 10^3 mm^4$ |
| Warping constant | $I_w = 309370 \cdot 10^6 mm^6$ |
| Partial factor (EN 1993-1-1 6.1 NOTE 2B) | $\gamma_{M0} = 1.0$ |
| Partial factor (EN 1993-1-1 6.1 NOTE 2B) | $\gamma_{M1} = 1.0$ |
Here are the properties of Steel S235
| E-modulus | $E = 0.21 \cdot 10^6 MPa$ |
| Yield strength | $f_y = 235 MPa$ |
Now, we are all set to design the steel beam🔥.
🕵️♂️ Cross-Section Classification
Steel cross-sections have to be classified in order to find out if the specific element can be calculated with plastic or elastic analysis.
EN 1993-1-1 5.5.2 (1) describes the differences between the 4 classes.
In order to find the class of a beam with cross-section IPE360 we need to follow the checks of EN 1993-1-1 Table 5.2. So let’s do that.
We differentiate between outstand flange and internal compression parts.
Internal compression parts (EN 1993-1-1 Table 5.2 (sheet 1 of 3))
When we look at the table, we see that for a IPE360 cross-section, we need to first define the parameters c and t.
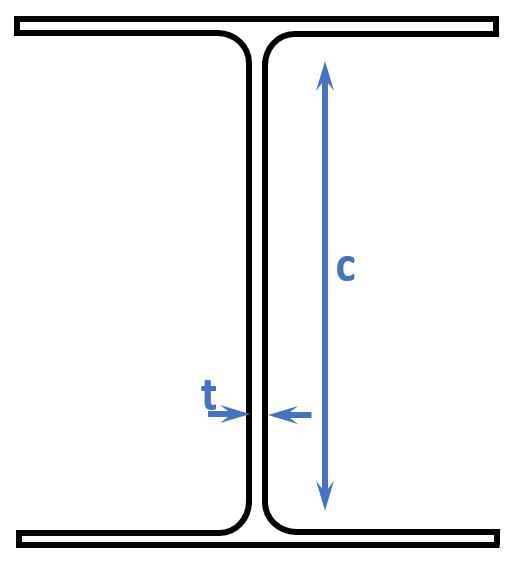
$$t = t_w = 8mm$$
$$c = h-2 \cdot t_f – 2 \cdot r = 360mm – 2 \cdot 12.7mm – 2 \cdot 18mm = 298.6mm$$
Now in the table we need to pick the 1st column because the beam (cross-section) is exposed to bending.
A column which is only exposed to an axial load would be the 2nd column because an axial load leads to compression in the cross-section.
$$\frac{c}{t} = 37.3$$
We can see from the table that if this value is smaller than $72 \cdot \varepsilon$ then the cross-section belongs to class 1. $\varepsilon$ is calculated as
$$\varepsilon = \sqrt{\frac{235MPa}{f_y}} = 1$$
Check
$$72 \cdot \varepsilon = 72 > 37.3$$
The internal compression parts of a IPE360 can therefore be calculated as Cross-section class 1.
Outstand flange (EN 1993-1-1 Table 5.2 (sheet 2 of 3))
As for the internal compression parts (the web), we need to define the parameters c and t for the flange classification.
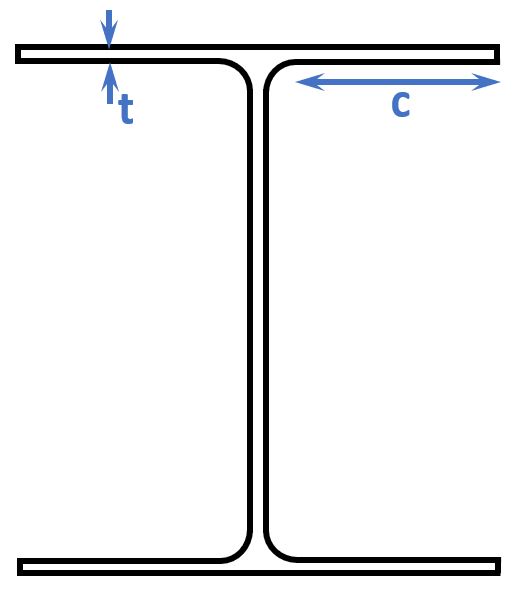
$$t = t_f = 12.7mm$$
$$c = \frac{b – t_w – 2 \cdot r}{2} = \frac{170mm – 8mm – 2 \cdot 18mm}{2} = 63mm$$
Let’s calculate the ratio again.
$$\frac{c}{t} = 4.96$$
Check
$$9 \cdot \varepsilon = 9 > 4.96$$
The outstand flange of a IPE360 can therefore be calculated as Cross-section class 1.
Overall can be said that in the design the plastic cross-sectional properties can be utilized.
➡️ Internal Forces
We need to calculate the max. bending moment and shear force before we can design the steel beam.
The formulas to calculate the internal forces of a simply supported beam exposed to a point load can be found from this post.
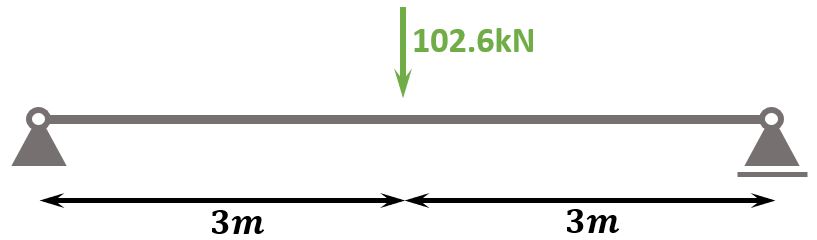
Max bending moment
$$M_d = 1/4 \cdot Q \cdot l = 1/4 \cdot 102.6 kN \cdot 6m = 153.9 kNm$$
Max shear force
$$V_d = 1/2 \cdot Q = 1/2 \cdot 102.6 kN = 51.3 kN$$
✍️ ULS Bending Moment Verification
In the ULS (ultimate limit state) Design we verify the stresses in the steel members due to bending, shear, normal forces and buckling.
However, since the beam is not exposed to axial loading, we don’t verify the column for tension or compression.
The bending design resistance is calculated with EN 1993-1-1 (6.13) as
$$M_{c.Rd} = \frac{W_{pl.y} \cdot f_y}{\gamma_{M0}}$$
with
$\gamma_{M0} = 1.0$ Partial factor found in EN 1993-1-1 6.1 NOTE 2B.
💡Note that the value of 1.0 is taken from the general Eurocode. This values might be defined differently in the national annex of your country.
$$M_{c.Rd} = \frac{1019 \cdot 10^3 mm^3 \cdot 2.35 \cdot 10^5 \frac{kN}{m^2}}{1.0} = 239.5 kNm$$
The biggest design bending moment is found from Load combination 3 as
$$M_{d} = 153.9 kNm$$
Utilization check
$$\eta = \frac{M_{d}}{M_{c.Rd}} = \frac{153.9 kNm}{239.5 kNm} = 64.27\%$$
The Cross-section is 64.27% utilized for bending.
👨🔬 ULS Shear Verification
The shear design resistance is calculated with EN 1993-1-1 (6.18) as
$$V_{pl.Rd} = A_v \cdot \frac{\frac{f_y}{\sqrt{3}}}{\gamma_{M0}} = 476.7 kN$$
Check if shear buckling verification is required (EN 1993-1-1 (6.22)).
$$h_w = h – 2 \cdot t_f = 334.6mm$$
$$\frac{h_w}{t_w} = 41.83$$
$$\eta = 1.0$$
Check
$$\frac{h_w}{t_w} < 72 \cdot \frac{\varepsilon}{\eta} $$
$$41.83 < 72$$ ✔️✔️
The check is verified and therefore no shear buckling verification is required.
Utilization check
$$\eta = \frac{V_{d}}{V_{pl.Rd}} = \frac{51.3 kNm}{476.7 kNm} = 10.7\%$$
👩🏫 ULS Lateral Torsional Buckling
The flanges aren’t attached or fixed continuously to, e.g. a roof. Therefore, lateral torsional buckling needs to be checked for.
We chose the static system of a simply supported beam which has a buckling length of
$$l_{cr} = 1.0 \cdot l = 6.0m$$
The elastic critical moment is calculated according to Designers’ guide to EN 1993-1-1 Eurocode 3: Design of steel structures (2005) (D6.10) as
$$M_{cr} = C_1 \frac{\pi^2 EI_z}{l_{cr}^2} \cdot (\frac{I_w}{I_z} + \frac{l_{cr}^2 GI_v}{\pi^2 EI_z})^{0.5} = 231.37 kNm$$
The value of $C_1$ is taken from Table 6.12 from Designers’ guide to EN 1993-1-1 Eurocode 3: Design of steel structures (2005).
$$C_1 = 1.365$$
💡All other parameters are defined in the table at the beginning of the post.
Non-dimensional lateral torsional slenderness (EN 1993-1-1 (6.56))
$$\lambda_{LT} = \sqrt{\frac{W_{pl.y} \cdot f_y}{M_{cr}}} = 1.017$$
Buckling curve from EN 1993-1-1 Table 6.4 as
$$h/b = 2.12 \mbox{ -> Curve b}$$
Imperfection factor (EN 1993-1-1 Table 6.3)
$$\alpha_{LT} = 0.34$$
From EN 1993-1-1 (6.57)
$$\lambda_{LT.0} = 0.4$$
$$\beta = 0.75$$
$$\Phi_{LT} = 0.5[ 1+ \alpha_{LT} (\lambda_{LT} – \lambda_{LT.0}) + \beta \lambda_{LT}^2] = 0.99$$
More buckling reduction factors are calculated with EN 1993-1-1 (6.49)
$$\chi_{LT} = \frac{1}{\Phi_{LT} + \sqrt{\Phi_{LT}^2 + \beta \lambda_{LT}^2}} = 0.69$$
But
$$\chi_{LT} < \frac{1}{\lambda_{LT}^2} \mbox{ and } \chi_{LT} < 1$$
$$0.69 < 0.97 \mbox{ and } 0.69 < 1$$✔️✔️
Design buckling resistance (EN 1993-1-1 (6.55))
$$M_{b.Rd} = \chi_{LT} \cdot W_{pl.y} \cdot \frac{f_y}{\gamma_{M1}}$$
$$M_{b.Rd} = 0.69 \cdot 1019 \cdot 10^6 mm^3 \cdot \frac{2.35 \cdot 10^5 \frac{kN}{m^2}}{\gamma_{M1}} = 165.0 kNm$$
And finally, the utilization check
$$\eta = \frac{M_d}{M_{b.Rd}} = 93.3\%$$
The Cross-section is 93.3% utilized for lateral torsional buckling.
📱 SLS Deflection Verification
The deflection of a simply supported steel beam exposed to a point load at midspan is calculated with this formula.
$$w_{max} = \frac{Q l^3}{48 E I_y}$$
In case you have a different static system or load, make sure to check out our beam deflection guide covering all formulas YOU need.
$$w_{max} = \frac{72 kN \cdot (6m)^3}{48 \cdot 2.1 \cdot 10^8 \frac{kN}{m^2} \cdot 1.627 \cdot 10^8 mm^4} = 9.48mm$$
Allowable deflection: EN 1993-1-1 7.2.1 states that the max allowable deflection must be agreed upon with the client and for each project.
So in this tutorial, we decide to use $l/200$ as the limit deflection.
$$\frac{l}{200} = \frac{6m}{200} = 30mm$$
Utilization check
$$\eta = \frac{w_{max}}{30mm} = 31.6\%$$
The deflection of the beam is 31.6% utilized. So finally all checks on the beam are fulfilled.👍👍
Once the bending, shear, lateral torsional buckling and the deflection criteria are verified, we successfully designed the beam.💯💯
If you are new to structural design, then check out more of our design tutorials where you can also learn how to design wood elements such as
But now, I would like to hear from you: Have you already designed a steel beam in university or at your work? And which semester was that in? Tell us a bit about the structure, as we all want to learn from each other.✍️
![Rafter Roof Design [Step-By-Step Guide]](https://www.structuralbasics.com/wp-content/uploads/2022/04/Rafter-Roof-Design-1-768x439.jpg)

![Reinforced Concrete Column Design [2024]](https://www.structuralbasics.com/wp-content/uploads/2024/02/RC-column-design-768x439.jpg)
![The Fink Truss [All YOU Need to Know]](https://www.structuralbasics.com/wp-content/uploads/2022/12/Fink-truss-768x439.jpg)
![Masonry Wall Subjected To Point Load [Step-By-Step Guide]](https://www.structuralbasics.com/wp-content/uploads/2024/03/Masonry-wall-subjected-to-point-load-768x439.jpg)