Uniformly Distributed Load [All YOU Need To Know]

UDL’s can be a difficult concept to understand for those who are not trained in engineering or physics.

Questions arise such as: 🙋‍♀️🙋‍♀️

How do engineers account for UDLs when designing structures? And why are UDL’s important to understand in the first place? Or how do you calculate the magnitude and distribution of a UDL?

In this blog post, we’ll break it down. 📝📝

We’ll show you, step-by step, what a uniformly distributed load is, how it’s visualized in engineering, real-world examples and much more.

So let’s get started. 🚀🚀

Definition – Uniformly Distributed Load (UDL)

A uniformly distributed load (UDL) is an action (load) on a structural element such as a beam, slab or column which has the same value at any point.

In general, there are

• uniformly distributed line loads and
• uniformly distributed area loads

Examples of this load would be snow, wind, live or dead load.

But an image explains it much better than words. Let’s have a look. ⬇️⬇️

Visualization of Uniformly Distributed Loads

The uniformly distributed load is represented as arrows of the same length, and the start and endpoints of the arrows are connected to each other.

The picture below shows a uniformly distributed line load.

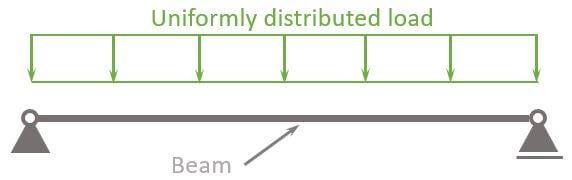
This connection is important. If there wasn’t any horizontal lines, the arrows would represent point loads.

The picture below shows a uniformly distributed area load on a slab, which is simply supported.

The length of the arrows is the same in any point.

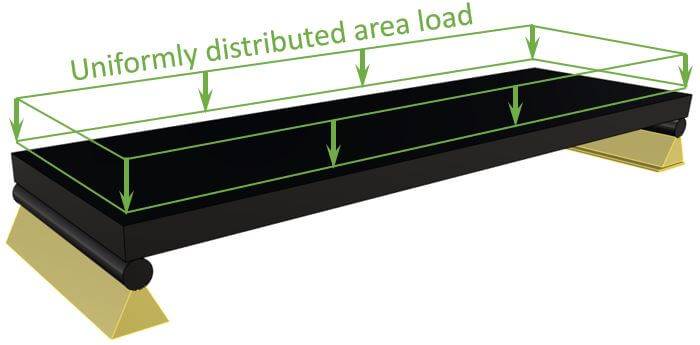
In a later section, we’ll transform an area load to a line load.

So stick with us!

Values and Units

Uniformly distributed loads have values and units, and based on these, the internal forces of the structural element are calculated.

Once the internal forces are known, the structural element can be designed (cross-sectional dimensions are found).

The unit of the UDL is kN/m² (kilonewton per square meter) for area loads and kN/m (kilonewton per meter) for line loads. This applies to Europe and anywhere where Eurocode is used.

In the US, kip/ft² (kips per square foot) for area loads and kip/ft (kips per foot) is used as unit.

The value at any point of the width of the uniformly distributed load is the same. Sounds complicated, I know. Let’s put those words into a picture.

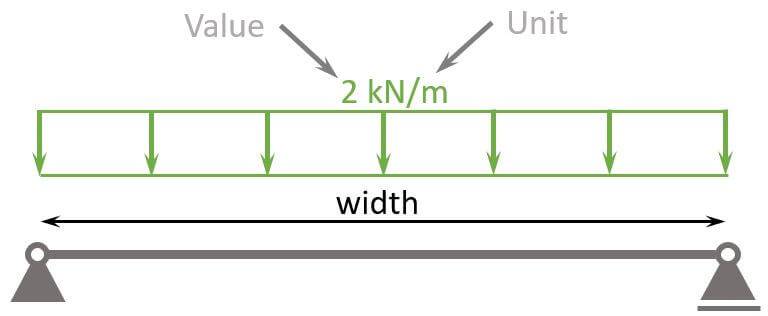
Real-World Examples of UDL

UDL’s can come from a variety of different load types, such as:

• Snow load
• Wind load
• Dead load
• Live load
• Earth pressure
• Water pressure

We have written an extensive article about loads on buildings, where we go into detail with every load type. So check that out if you want to learn more.

Now, let’s look at some practical examples.

Snow load on secondary beams of a timber flat roof

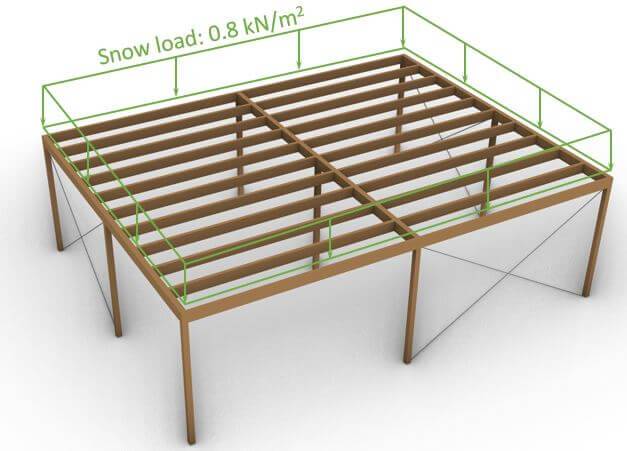
The snow load on this flat roof is an example of an area UDL but also a line UDL, because the area load (kN/m²) is transformed into line loads (kN/m) which are applied on the timber beams.

We’ll show in the next section how to convert area loads into line loads.

You can find a full structural guide on how to design this timber roof here.

Design load on the bottom chord of the pratt truss

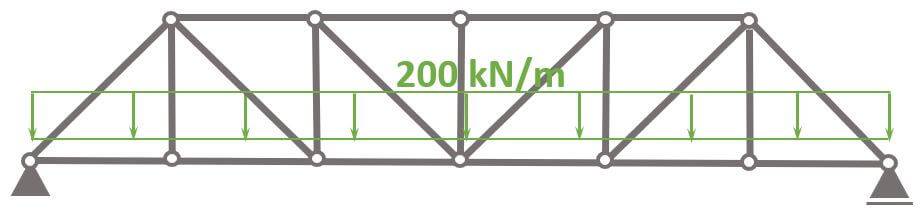
The uniformly distributed design load of 200 kN/m is applied on the bottom chords of the pratt truss, which is mostly used for bridge structures.

This design load is the result of a load combination of traffic and dead load.

You can check out our calculation guide for pratt trusses here.

Now, let’s have a look at how we convert area loads into line loads.

Transformation of Area Load to Line Load (UDL)

Let’s run through the example where a UDL area load of 2 kN/m² is applied to a slab.

The slab is supported by 2 beams which have a distance of 5 m.

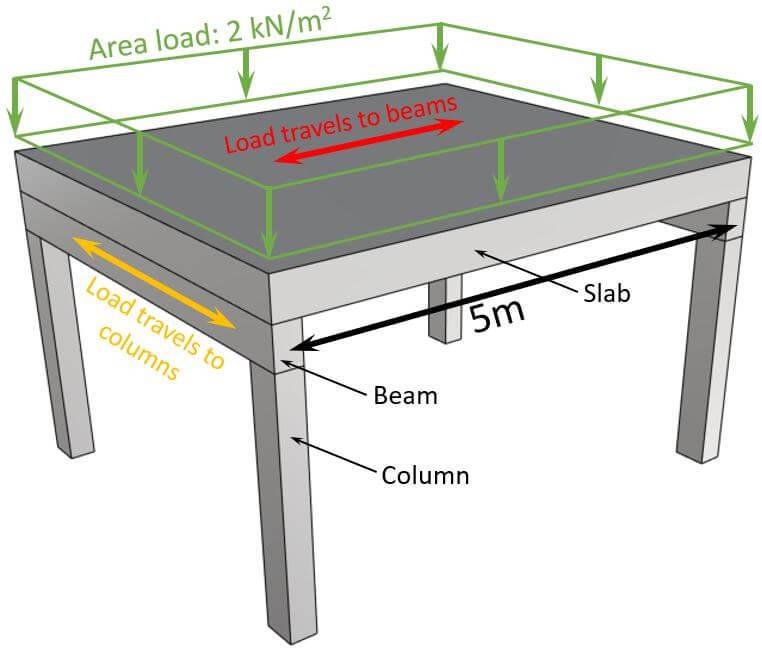
To calculate the UDL line load that you can apply on 1 of the beams, we multiply the area load with half of the distance of the 2 beams.

$$2 \frac{kN}{m^2} \cdot \frac{5m}{2} = 5 \frac{kN}{m}$$

Conclusion

Now, that you got an understanding of uniformly distributed loads, you can learn about the other loads that act on beams.

Once you are confident with the different types of loads, you can calculate the reaction and internal forces.

Then, finally, structural elements can be designed with different materials based on the internal forces. Check out our structural guides. 👇👇

• Structural design of a purlin roof
• Steel beam design
• Structural design of a timber beam

I hope that this article helped you understand uniformly distributed loads. In case you still have questions, let us know in the comments below. ✍️✍️

Uniformly Distributed Load FAQ